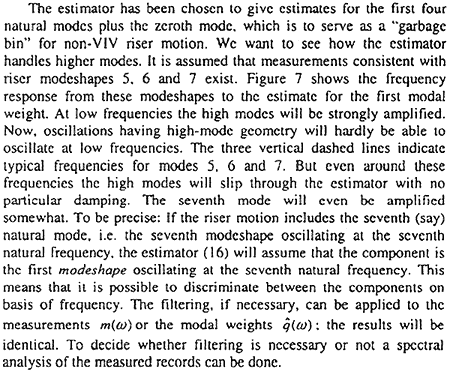
Consequently the latter is weighted less by the estimator at high frequencies. As mentioned above, the first mode is not likely to appear in the whole frequency range shown by the figure. Again, the vertical dashed line indicates a likely frequency of the first mode.
MODE LEAK
AN ANALYSIS EXAMPLE
The Helland-Hansen data consists of than 916 recorded sets of riser motion and current. Each record of riser measurement has a length of 34 minutes and consists of 4096 samples. From this amount of data 357 sets were selected for analysis. A closer description of this analysis and the results are given by Kaasen et al. (2000). In what follows the method for analysing and identifying VIV is exampled by one case. This case is a typical lock-in case, with an almost pure 2nd mode VIV.
The dominant part of VIV takes place in a plane that is normal to the direction of the current. For presentation of results therefore, the X and Y components of the riser motion were transformed to axes coinciding with the in-line and cross-flow directions as determined from the current profile (not shown). Using the estimator described above, modal weights and displacements were estimated. Before the estimator was applied, all records of measurement were filtered in the frequency domain using a sharp band-pass filter with cutoff frequencies 0.01 Hz and 0.16 Hz. The bandpass range coincides with the expected frequency range of the selected modes (1-4).
For the six instrumented locations on the riser, Figure 8 shows the estimated displacements in the cross-flow direction in the form of time traccs and amplitude spectra (note: not power density spectra). As can be seen the 2nd mode dominates completely, but there are some irregular low frequency action to be seen and some blurred energy around the third natural frequency. The low frequency motion is most prominent toward the top of the riser indicating that its source is the slowly varying motion of the dynamically positioned drilling vessel.
The estimated modal weights (modes 1-4 plus mode 0) are shown in Figure 9. The result demonstrates that the estimator works well (at least for the case shown). Mode 2 clearly dominates. The bulk of vessel-induced low frequency motion is picked up by the zeroth ("garbage") mode, as intended. Still, there are some small amounts of mode 2's frequency to be seen on the other mode estimates. The probable reason for this is that the modeshapes used in the analysis impossibly can be one hundred percent correct, since they are calculated numerically from a linear model with some uncertain parameters.
Using the estimated modal weights (participation factors) and precalculated modeshapes the rms profile of the riser is calculated and shown in Figure 10. In this calculation the zeroth mode was excluded, since it is not a VIV motion. The figure shows clearly the dominance of the second mode.
The effect of neglecting the gravitational disturbance is also demonstrated in Fig. 10. The dotted rms-profile is calculated without taking the g-influence into account. The difference from the true profile clearly shows the importance of including the gravity in the model.
CONCLUSION
A method for estimating the VIV displacement of a marine riser from full-scale measurements of acceleration and rate of rotation is developed. A fundamental problem with using accelerometers to measure riser motion is that the signals may be significantly influenced by gravity due to the angular motion of the riser. On the other hand, it is this gravitational "disturbance" that makes detection of low frequency motion possible. The estimation method can handle the influence of gravity utilising a modal approach in combination with a least-squares technique. Also the method can deal with cases where different instrumentation is used in different locations on the riser (i.e. not every instrument container included a rotation rate device). The estimator operates in the frequency domain, which is efficient from a computational view. The estimator uses precalculated modeshapes, which must be derived from a theoretical model of the riser. An example shows that the estimator works well. The error of neglecting the influence of gravity can be large, as is demonstrated.
ACKNOWLEDGEMENT
The work described in this paper was performed as a part of the Norwegian Deepwater Programme (NDP). Partners in the NDP group have been BP Amoco, Conoco, Exxon, Mobil, Norsk Hydro, Saga, Shell, and Statoil. The author thanks CorrOcean Robit Technology AS for permission to use Figure 1.
REFERENCES
Olufsen, A and Garside, R (1999). "Riser & Mooring Research Activities Within the Norwegian Deepwater Program. "Deep Offshore Technology Conference, Stavanger, Norway.
Halse, KH (2000). "Norwegian Deepwater Program. Improved VIV Prediction, "Paper 11996, Offshore Technology Conference, Houston, USA.
Kaasen, KH. Lie, H. Solaas, F, and Vandiver, JK (2000). ). "Norwegian Deepwater Program. Analysis of Vortex-Induced Vibrations of Marine Risers Based on Full-Scale Measurements." Paper 11997, Offshore Technology Conference, Houston, USA.
Goodwin, GC and Payne, RL (1977). "Dynamic System Identification Experiment Design and Data Analysis. "Academic Press, New York.
Cornut SFA and Vandiver JK (2000). "Offshore VIV Monitoring at Schiehallion - Analysis of Riser VIV Response." Paper 5022, ETCE/OMAE 2000 Joint Conference. New Orleans, USA.
Gopalkrishnan, R (1993). "Vortex-Induced Forces on Oscillating Bluff Cylinders." Doctoral Thesis, Dept. of Ocean Engineering. Massachusetts Institute of Technology and Woods Hole Oceanographics Institution, USA.