where α = T_m / T_e.

Thus, from the above relation, it makes clear that the success ratio p is controlled by the two elements, ie, T_e, and T_m. Here if α = 1, p is 1/2, which means T_e = T_m. Therefore, it can be found that for succeeding in the faiult diagnosis, the parameter α is better to be small as possible. It is very important that T_m is smaller than T_e, or in other word, it is better that p is very close to 1.

　

2.3 Description of model [2]

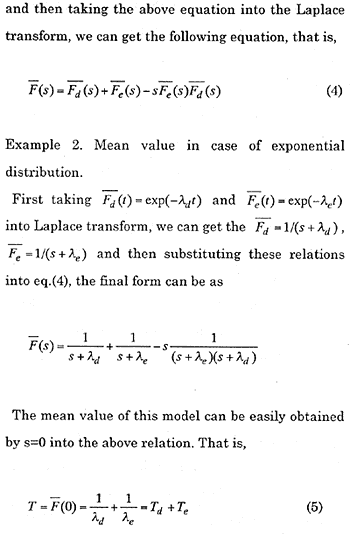
(1) Basic maintenance model: It is the case where maintenance can be done in the system failure only, ie, CM. Its failure distribution F(t) is given by the following equation:

　

　

　

(2) Model A: In this model, it is possible to find defects/faults in the system instantly by monitoring before the system fails. T_va and _wa mean the expected time interval of CM and PM respectively in this model, and these theoretical results are represented as

T_va =T_d / (1-p)+T_e

T_wa = T_d / p +(1/p - 1) T_e　(6)

(3) Model B: This model requests time to diagnose defect/fault. The expected time interval of CM T_vb and the expected time interval of PM T_wb are given by the following relations:

T_vb =T_d / (1-p)+T_e

T_wb = T_d / p +(1/p - 1) T_e　(7)

Therefore, eqs. (6) and (7) are same relation each other.

BACK　　　CONTENTS　　　NEXT