¹Nihon University, College of Industrial Technology

Narashino, Chiba, JAPAN

c03810@cit.nihon-u.ac.jp

　

²Ark Information System, Tokyo, JAPAN

³Columbia University, Lamont-Doherty Earth Observatory, New York, USA

　

　

As one of measures for controlling the emission of carbon dioxide (CO₂) into the atmosphere, attention is focused on making effective use of the ability of the ocean to absorb CO₂, and an evaluation is performed on the ability to isolate CO₂ from the atmosphere by emitting CO₂ into areas deeper than the middle layer of the ocean. The subarctic region of the northern Pacific Ocean has recently been recognized as a sea area, which has the potential to absorb CO₂ although oceanographical data of this region are comparatively scarce except for sea areas adjacent to Japan, the Aleutian Islands and the Canadian coast. As this sea region is thought to play decisive roles in the climate fluctuations of the world in the time-scale of decade's years, it is indispensable to correctly evaluate effects of the ocean sequestration of CO₂ when this technique would actually be undertaken. Based on the flow fields of the whole Pacific Ocean, which were calculated by the data assimilation system, the authors examined changes in CO₂ concentration of the ocean, which may result from CO₂ introduction into the subarctic zone of the northern Pacific Ocean.

　

　

The global warming, ascribed to increase in greenhouse gas emission by human activities, has become one of the most serious environmental problems on our planet. The Third Conference of the Parties (COP3) to the U.N. Framework Convention on Climate Change, which was held in 1997 in Kyoto, Japan, adopted targets of the cut of greenhouse gas emission form the 1990 level for major industrial countries. The target for Japan was a 6% cut by 2008. Under these circumstances, a greater amount of attention has recently been given to the technique of ocean sequestration of carbon dioxide (CO₂), one of the main greenhouse gases. The CO₂ ocean sequestration technique consists in directly introducing the man-made CO₂ and dissolving it in seawater of the deeper layers of ocean. As CO₂ injected into seawater of the deeper layers of ocean may eventually be dispersed into wider regions of the sea owing to current and diffusion, so it is necessary to examine the flow field of the ocean. Numerical simulation techniques using ocean circulation models have been widely employed in complementing for shortcomings of actual observation. Bryan (1969a) was the first to introduce for this purpose the Primitive Equation Systems, which has influenced almost all Ocean General Circulation Models (OGCM) until now (Hasumi, 2001). Accuracy of numerical simulation results is an important factor in estimating the effectiveness of CO₂ ocean sequestration. We used the method of Sarmiento and Bryan (1982), in which differences between observed and calculated values are corrected at each time step of calculation, thus enabling to simulate a realistic flow field based upon observation results. Instead of using the wind stress at sea surface calculated from buoy and shipboard data by the method of Hellerman et al. (1983), which has been adopted in many of OGCM, we utilized the satellite observation data of wind speed (Special Sensor Microwave/Imager: SSN/1) that were converted to wind stress by the Bulk equation. Finally, we examined the feasibility of ocean sequestration technique for a case in which the CO₂ in surplus of the emission cut targeted for Japan in COP3 was introduced into the Pacific Ocean, and discussed a proper regions of water into which CO₂ should be introduced if the object of CO₂ sequestration is to be attained.

　

　

　

Calculation covered the entire Pacific Ocean. The calculation mesh in the horizontal direction was longitude-latitude 2°x 2°(110E〜70W, 60N〜74S). We assumed eleven vertical layers as shown in Table 1. The coordinate system is spherical coordinate to horizontal direction. The equations used are equation of motion (1), hydrostatic approximation equation (2), continuity equation (3), equation of heat conservation (4), salinity conservation equation (5) and condition equation (6).

　

　

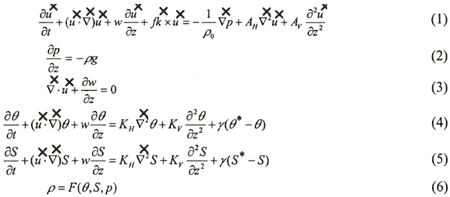
the horizontal flow velocity the horizontal gradient operator and ² the horizontal Laplacian operator of spherical coordinate, 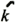u nit vector of triaxial reference system, w the vertical flow velocity, p is pressure, ρ is density, θ,θ^* the calculated value of potential water temperature and observed value of potential water temperature, respectively, S,S^* the calculated value of salinity and observed value of salinity respectively, f the Coriolis parameter, g the acceleration of gravity, γ the corrected term. AH the coefficient of horizontal eddy viscosity (1.0 x 10⁷cm²/s), A_v the coefficient of vertical eddy viscosity (1.0 x 10² cm²/s), K_H the coefficient of horizontal eddy diffusion (1.0 x 10⁶cm²/s) and Kv the coefficient of vertical eddy diffusion (1.0 x 10 cm²/s), its value is set up based on the value that Wada et al. (1996) used for calculation.

　

　

　

We introduced a term that assimilates calculated value and observed value in the salinity conservation equation and heat conservation equation after Sarmiento and Bryan (1982).

　

　

γs the sea surface value (1/50 days=2.31 x 10^-7 sec^-1),γD the value in deep water (1/250 day=4.6 x 10^-8 sec^-1), z the calculated depth, h the influenced depth of assimilation terms (=500 m).

　

By equation (7), observed data are assimilated continuously to a model through the assimilated term. When a calculated value deviates from an observed value, the assimilated term becomes bigger and works to return the calculated value to observed value (Fujio et al., 1992a, 1992b).

　

　

Wind stress was calculated from satellite observed data sets (SSM/I: (1988〜1998)) (Atlas et al., 1996) and the data were applied to a model. For calculation of wind stress, a bulk method was adopted (Garratt, 1997). This method evaluates the flux using the observation values at optional height on water surface. The flux calculation formula is given below:

　

　

ρ_a the air density, |V| the absolute value of wind speed, u_a and v_a are East-West and South-North components of wind speed (wind speed value measured at 10m high is used). C_D the Bulk coefficient (conversion coefficient for kinetic momentum) estimated by Kondo (1975). Generally, the value of C_D is determined by wind speed or by a function of wind speed and stability. As there are areas where tropical (10°S 120°E, 120°E 170°E) and sub-tropical (30°N, 35°S) area winds converge and have small value, it was necessary to meticulously define a bulk coefficient at breeze. As for water temperature and salinity data, we used data in the area between 100°E〜60°W, during about 80 years (1906〜1988), stored by JODC. For sea bottom topography are used the General Bathymetric Chart of the Oceans (GEBCO) published by the Canadian Hydrographic Service.

　

　

　

Figure 1 shows the calculated horizontal current field (water depth 10 m) after 30 years. The Northern Equatorial Current, the Kuroshio, California Current and the clockwise North Pacific sub-tropical circulation are reproduced. In the southern hemisphere, the South Equatorial Current, the Antarctic Circumpolar Current, the East Australia Current and the counter-clockwise South Pacific sub-tropical belt circulation are reproduced. In addition, detailed examination of the numerical results shows that the Equatorial Countercurrent, the Oyashio and the Subtropical Counter current are also well reproduced.