Then the pressure at the node is set to be the vapor pressure. The change of the vaporous cavity volume (or the void fraction at the node) is calculated by the oil velocities of both side of the cavity. When the cavity volume goes down below zero, the reunion of the oil column is assumed.

2.3 Fluid transients after a sudden coupler detachment
The case where the movement of the ship is so fast that the coupler is reluctantly cut off while the valves are still on the way of closing is also estimated as follows.
The flow state at the valve after the coupler detachment is classified into the following three cases.
(1) Oil flows out
(2) Air flows in
(3) Air (without oil vapor) flows out
When air exists at the downstream of the valve, the state is decided by solving the following equations simultaneously.
(i) Waterhammer compatibility equation by the method of Characteristics
(ii) General gas law
(iii) Pressure head - flow rate relation at the valve
With regard to (iii), the equations to be used are different depending on whether it is inflow or outflow, subsonic flow or sonic flow. Therefore, the simultaneous equations should be solved in cyclic way, by checking the proper equation of (iii) at each calculation. The calculation process is mainly based on the reference [1].
When the amount of air in the pipe is small, the temperature is presumed to be the same as the oil temperature. Tamb. The air follows the isothermal law approximately. However when the amount is large, the law is not valid any more. On the other hand, if compared with the case of adiabatic change, in actual situation the air is cooled by the ambient oil when it is compressed, and is heated when it is expanded. Consequently, the temperature of the air would be between Tamb and that of adiabatic change. Therefore, in order to estimate the actual change, calculations based on the isothermal change and adiabatic change are conducted.
3. RESULTS AND DISCUSSION
3.1 Influence of the oil head of shore tank on waterhammer pressure rise
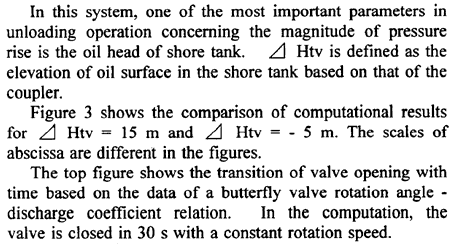
The middle figure shows the void fraction at the downstream of the valve ((A) in Fig.2). The length of computational reaches is 200 m in this case. Therefore, the void fraction of 0.1 corresponds to the cavity of 20 m.
Fig. 3 Pressure transient by constant rotation speed valve closing