The difference between our model and the linear model is clear; the linear model does not cotain advection of momentum so that much of our dynamics is not included in it. Consequently, the fact that our analytical prediction for the 1982 El Nino is not that different from the linear prediction is fortuitous. The difference between our model and the Gent and Cane model is less clear than the difference discussed above because the Gent and Cane model does include advection of momentum. It is probably due to the absence of friction (in our model) which is expected to increase the form-drag exerted (on the pool) and slow the pool down. Other possibilities are the neglect of motions in the lower layer, and the neglect of recirculation.
(c) The model sensitivity
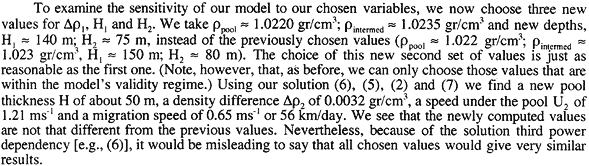
(d) Relationship of predicted speed to the observed speed
We just saw that our nonlinear speed is roughly 25% greater than the observed speed. This can be a result of the model weaknesses or a result of the way that the data have been handled (or both). We shall first discuss the modeling aspects that can lead to such a difference. As already alluded to, it is possible that the difference is due to the absence of induced motions below the intermediate layer in our model. These motions may exert additional form-drag on the pool and slow it down. Another possibility is that the difference is due to the fact that our model is inviscid and does not include breaking waves. As in the classical two-layer intrusion (Benjamin 1968), one would expect that, in reality, there would be some sort of breaking waves on the interfaces. These waves cause dissipation and, hence, prevent the Bernoulli from being conserved in the manner that we employed. Due to the reduction of energy, a reduction in the propagation speed is also expected to take place. A third possibility is that there is some recirculation (neglected in our computations) which, as mentioned earlier, slows the pool's propagation down.
An equally reasonable possibility (unrelated to the model) is that the actual migration speed along the equator is greater than the quoted observed values because of the averaging (over a band bounded by 4°N and 4°S) that the observational analysis (i.e., the solid line in Fig. 4) contains. As pointed out earlier, the pool is expected to behave like a forever-thinning tongue with the nose along the equator moving faster than off-equatorial regions. That is to say, in a similar fashion to the propagation of gravity currents along a solid wall on an f-plane, the thickness of the advancing current decreases as one moves away from the equator (wall) until it ultimately vanishes several Rossby radii away. Since the forward speed is proportional to the thickness, this implies that the propagation rate also decreases away from the equator suggesting that the averaging “hides” high propagation rates along the equator.