The results revealed that flashing of high-pressure saturated water and a subsequent water hammer occurred under specified experimental settings. The pressure peaked when steam generation or flashing occurred at the wall surface and when the flashing steam condensed. The pressure peak increased with the initial pressure of high-pressure saturated water. The magnitude of pressure peak was represented by Eq. (1). After the peaks, the pressure oscillated and reached equilibrium condition in a short time. The oscillation of the pressure might have been caused by a balancing action between the flashing of high-pressure saturated water and condensation of steam generated by the flashing in low-pressure, low-temperature water. To check the results of the experiments, experiments and numerical analysis where conducted for a case of saturated-pressure water discharging into an air field. The test geometry was similar to a classical test case of fast depressurization of a horizontal pipe initially filled with subcooled liquid (Edward's pipe). The results of the experiments and analysis showed that no pressure peak occurred when saturated high-pressure water was discharged into the air field.
The acquisition of hydrothermal data on the phenomena will be indispensable for the detailed design and development of heat plants or reactors for marine use.
APPENDIX.
A.SIMULATION OF FLASHING HAMMER PHENOMENA CAUSED BY LIQUID-LIQUID CONTACT CONDITION
As for growth and annihilation of bubble in pool, it is theoretically explained by well-known Rayleigh's solution with an inertial controlled bubble, or by Plesset-Zwick's solution with a thermal controlled bubble. On the base of the two solutions, Mikic et. al [14] have proposed an approximation solution with an energy controlled bubble. In this model, the flashing bubble growth on high pressure saturated water under depressurization is supposed to be governed by Mikic's solution. The generated pressure when in contact between high pressure saturated water and cold water was evaluated using a simple one dimension model shown in Fig. A-1. The assumptions used in the simulation are follows:
1) Temperature and pressure of the inertial layer are constant.
2) Temperature and pressure of the expanding layer are uniform.
The governing equations to simulate the flashing hammer phenomena are as follows The Mikic's solution are shown by Eq. (A-1)-(A-3)
Where Ja is the Jacob number.
Next, the steam generating ratio F, mass ratio of generating vapor bubble to that of the expanding layer, is defined as shown in Eq. (A-4). It was confirmed through the experiment, that the rate of bubble growth around the wall surface may be affected not only by its own thermal energy of the high-pressure saturated water, but also by heat supply from the wall on high-pressure saturated water-side. In order to investigate this effect, a small amount of the heat supply from the tube wall is added to Eq, (A-6) as a corrector + ΔF.
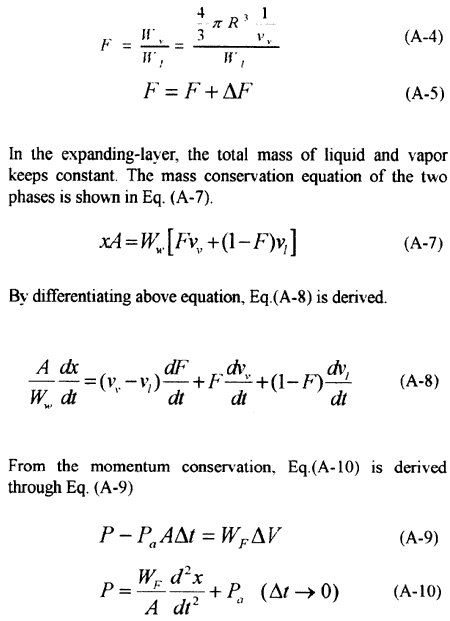
In calculation, a bubble radius under depressurization is evaluated by Eq's. (A-1)-(A-4). The steam generating ratio F is calculated by Eq, (A-5) using obtained bubble radius. Eq, (A-8) and (A-10) are explicitly by the finite differential method. Physical properties of the two-phases were evaluated by one step. In explicit numerical calculations, the result can be more stabilized as smaller time step. The simulations were conducted with various time steps, 10-6〜10-4s, to check the effect of time step on the result.
B. SIMULATION OF SATURATED WATER IN TUBE DISCHARGING INTO AIR FIELD (EDWARD'S PIPE)