Fig. 4 Lumped masses of diesel engine
3. X -MODE VIBRATION CONTROL BY DYNAMIC VIBRATION ABSORBER
In general, dynamic vibration absorber has been one of the classical method to control vibration specially for small machinery. However, application for 2 stroke diesel engine relatively large structure was issued by Mitsui Eng. & shipbuilding co. Ltd. (6) In this chapter, X-mode vibration control by using dynamic vibration absorber for 30 MW diesel power plant adapting 9K80MC-S engine is theoretically verified and also confirmed by actual vibration measurement.
In using dynamic vibration absorber, it is more effective if major resonance is within engine operating speed range and also equivalent mass of engine column is relatively small such as X-mode vibration. It is convenient for designing dynamic vibration absorber to convert complex lumped mass model shown on Fig. 4 into one mass equivalent model. Vibration mode at node i, j may be determined by FEM modelling method or vibration measurement data for similar project and equivalent mass of X-mode vibration can be calculated using Eq. (1). Also equivalent damping (ce) and equivalent stiffness (ke) can be found out in the same way.
X-moment generated from engine is calculated using cylinder combustion pressure and torque harmonics considering inertia force of reciprocating masses. And for X-mode vibration estimation, radius of gyration for crankshaft and length between cylinder and dynamic vibration absorber are furtherly considered and equivalent mass-spring system can be expressed as Eq. (2).
The equivalent model for 2 degree of freedom vibration system adapting dynamic vibration absorber to engine is shown on Fig. 5 and equation of motion can be expressed as Eq. (3), (4) by disregarding hysteresis damping of dynamic vibration absorber inner spring and absolute damping of foundation for easier calculation.
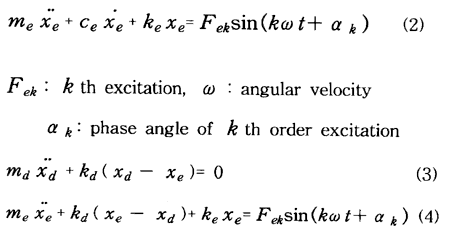
Vibration amplitudes of engine and dynamic vibration absorber are found out as Eq. (5), (6) derived from Eq. (3), (4). For exact tuning, natural frequency of engine and dynamic vibration absorber should be same which means ωd is satisfied with Eq. (7).